As we travel around the country, we are excited to see that fluency has been elevated in school-based discussions and has drawn the attention of many teachers. Done correctly, the development of numerical fluency empowers students and helps them develop lifelong understanding and confidence. Done wrongly or poorly, we deprive students of mathematical empowerment and send them messages that severely undermine their long-term mathematical development. We see exceptional teachers who are fully committed to teaching conceptually, but who still resort to speed tests because they see no alternatives. We see good mathematics programs that fail to give teachers structures for instruction or effective strategies that develop fluent mathematicians. And the questions we get remain essentially the same:

• "How do I teach number facts so that students know their facts with fluency?"
• "How do I help students who only want to follow rules that they obviously don't understand to compute accurately, efficiently and with confidence?"
• "Why do my students have such difficulty estimating sums, difference, products, and quotients?"

We believe that the core of the problem is that most teachers today learned mathematics as routines and procedures. When asked for the sum of eight and five, they have no problem answering “thirteen,” and explaining that this is simply a “fact I have memorized.” But when asked to apply this knowledge to 38 + 5, these same teachers ask for pencil and paper and resort to procedures including “and carry the one.” These teachers (and many of our students) do not have numerical fluency. They know their facts, but have not acquired the critical ability or disposition to think and reason numerically in ways that represent the fluency called for by the Common Core. Simply put, the facts are essential, but not enough.

In addition, many teachers are the products of instruction that made regular use of timed tests of facts. They struggle to find other, more effective, ways to build students’ fluency. Unfortunately, this keeps the focus on rote memory and speed, not on understanding and the powerful thinking and visualization strategies that undergird true fluency. As a result, far too many students, early in their mathematical careers, learn that mathematics is arithmetic and that arithmetic is the mindless memorization and regurgitation of facts and procedures
that don’t have to make sense and that don’t relate to the bigger aspects of conceptual understanding.

Instead, we envision teaching that starts with that conceptual understanding first, and builds fluency from it.

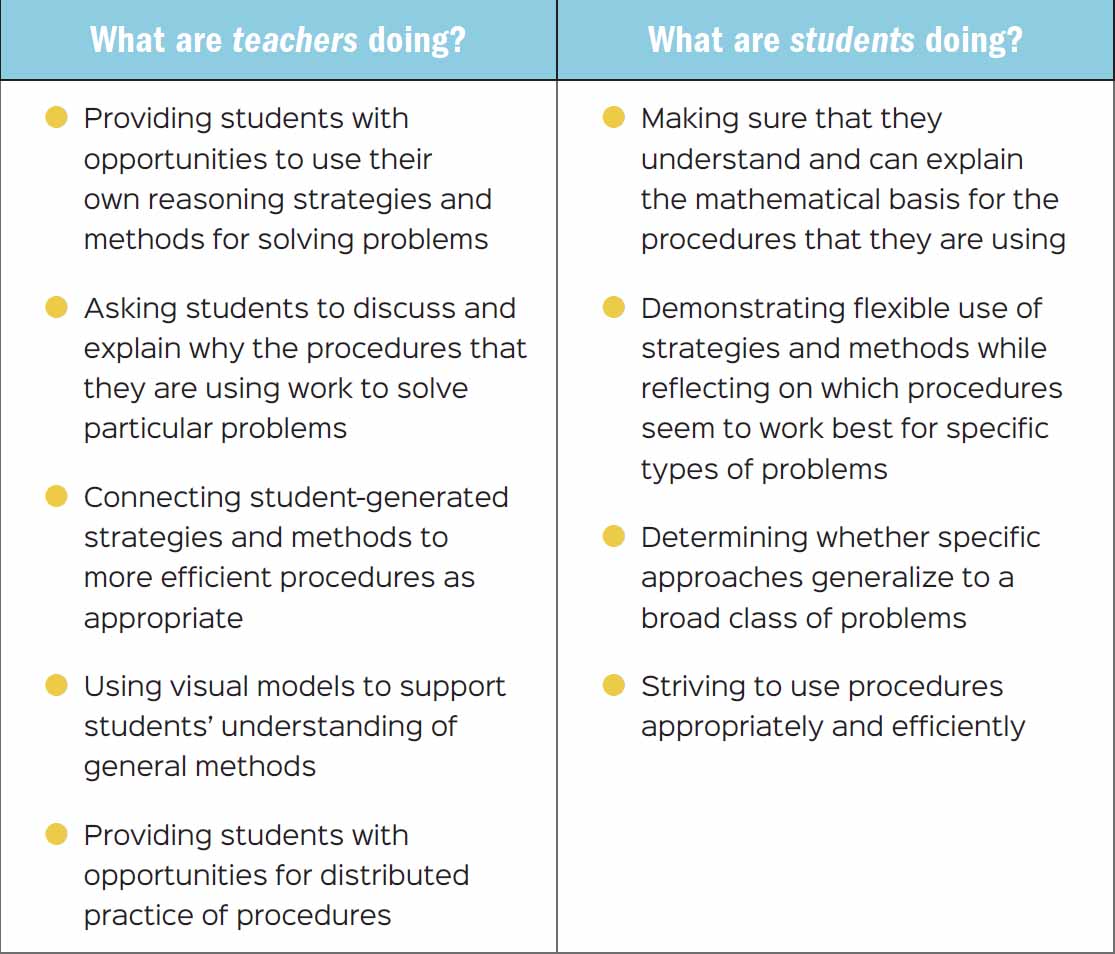Build procedural fluency from conceptual understanding (NCTM 2014, 47-48)

There is an unacceptable chasm between traditional mathematics instruction, that rarely works for more than one-third on our students, and this kind of mathematics instruction, that truly empowers nearly all students.

…

Learn more about Developing Numerical Fluency at Heinemann.com

Follow us on Instagram @heinemannpub to stay up to date on the latest books, your favorite authors, and upcoming events!

Patsy Kanter is an author, teacher, and international math consultant. She worked as the Lower School Math Coordinator and Assistant Principal at Isidore Newman School in New Orleans, Louisiana, for 13 years. Patsy is the co-author of Every Day Counts: Calendar Math and a consulting author for Math in Focus. You can follow her on Twitter @patsykanter

Steve Leinwand is the author of the bestselling Heinemann title Accessible Mathematics: Ten Instructional Shifts That Raise Student Achievement.He is Principal Research Analyst at the American Institutes for Research in Washington, D.C., where he supports a range of mathematics education initiatives and research. Steve served as Mathematics Supervisor in the Connecticut Department of Education for twenty-two years and is a former president of the National Council of Supervisors of Mathematics.