
The current reality in education is that as teachers we have a lot to teach in a short period of time. It is not feasible to give up large chunks of math instruction in order to prepare students for math workshop. Rather, minilessons are designed to complement your math instruction.
Overview
In this lesson, students think about the importance of exploring math in more than one way.
Many of us were taught that in a math problem, there is one—and only one—answer. And often we were taught to believe that there is only one way to solve the problem as well—the teacher’s way.
The beauty of math is that, with problems that have just one correct answer, there can be many ways to arrive at that answer. And with open-ended problems, there can be many correct answers! As teachers, we should respect different strategies—students who continually find alternate ways to solve problems are rewarded with a greater knowledge of number sense and problem-solving. As teachers, we should also challenge students to find all the answers—not just one.
It is critical to do this lesson within the first few days of starting math workshop. It is even more important to remember to include tasks like this in the days that follow.
Materials
- sticky notes
- an open-ended problem (an age-appropriate task that has multiple entry points and multiple answers; see suggestions at the end of this lesson)
Directions
Math is such an interesting subject. Many people like it because they like to find a “right” answer. However, many don’t realize that there are often several “right” answers or many methods for reaching a correct answer.
Show students this image:
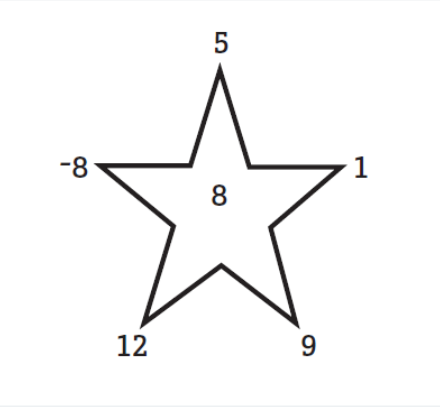
Think about the following problem: manipulate the numbers in the corners of the star using any operations to achieve the middle number. (Feel free to adjust this problem to fit students’ needs.)
Now write down one way to get the answer “8.”
Once every student is ready, ask students to share their responses with their group. Then ask every group to share two ways out loud to the whole class. Record the equations where everyone can see them.
Equations for the problem, __________= 8 using the digits above, may include
- 12 − (5 − 1) = 8
- 92 − (-8)2 − 9 = 8
- (12 × -8) // (-8 – 5 +1) = 8
I am now going to challenge you to work with your group to come up with as many other correct ways as possible. You can use parentheses, exponents, multiplication, division, addition, subtraction, or a combination of these operations. Try to use more than two numbers.
Give students some time to generate a list of possibilities. Then have students share their groups’ ideas again, and write the equations where everyone can see them. Have students talk about how each one is correct. Reflect on the fact that there is more than one correct “answer.”
So, each group was correct. Can you imagine if we had stopped at 12 − (5 − 1) = 8? If we had stopped there, so much thinking would not have happened. So much learning and so many great ideas would not have been shared!
I appreciate that we’ve been respectful of one another’s ideas. Even if someone used a different method or had a different idea than you, this does not mean that they are wrong. And even if their way does not work out, we can still learn from that as well. You have shared with me some ideas that I had not thought of for this problem!
SUGGESTED OPEN-ENDED PROBLEMS
Here are a few ideas for open-ended problems to use with this lesson and others. One of our favorite resources for these kinds of problems is the Good Questions series by authors Peter Sullivan, Pat Lilburn, Lainie Schuster, and Nancy Canavan Anderson.
- Francine is putting a fence around her flower garden. She has 36 feet of fencing for her garden. What might be the area of her garden?
- The area of Harry’s herb garden is 20 square feet. What might be the perimeter of the garden?
- Rachel’s piggy bank has 11 coins. How much money might be in Rachel’s piggy bank?
Wally, Zola, and Yasmine together have 56 toy cars. Wally has more cars than Zola. Zola has 5 fewer cars than Yasmine. How many toy cars might each child have?



